How To Eliminate Cyclical Unemployment

Background (Standard Econ)

When a recession hits, it causes unemployment. This fact is so obvious few people stop to wonder why.

However, for someone with introductory knowledge of economics, this empirical reality should actually be confusing. According to Econ 101 theory, competitive markets shouldn't have shortages unless the government implements a price floor: if there is unemployment, wages should just fall until the unemployed find jobs.

Now, admittedly, some of the people laid off during a recession were working minimum wage jobs. For them, the "price floor" story is probably correct. However, the vast majority of those laid off are being paid above the minimum wage. What gives here?

The answer is sticky wages.

In brief, cutting wages is hard on everyone (to different degrees):

• workers - who get paid less
• managers - who have to be the bad guy and hurting people they spend hours each day with (while also likely getting a pay-cut, themselves)
• owners - who have to suffer the reduced output that comes from having angry/demotivated workers and managers

However, if inflation is 2%, then wages get cut 2% each year by default (in real terms). Workers, managers, and owners are all much less upset with this scenario.

In short, this means cutting real wages is done all the time while cutting nominal wages happens only in dire situations.

This isn't just theorizing. The data backs this up:

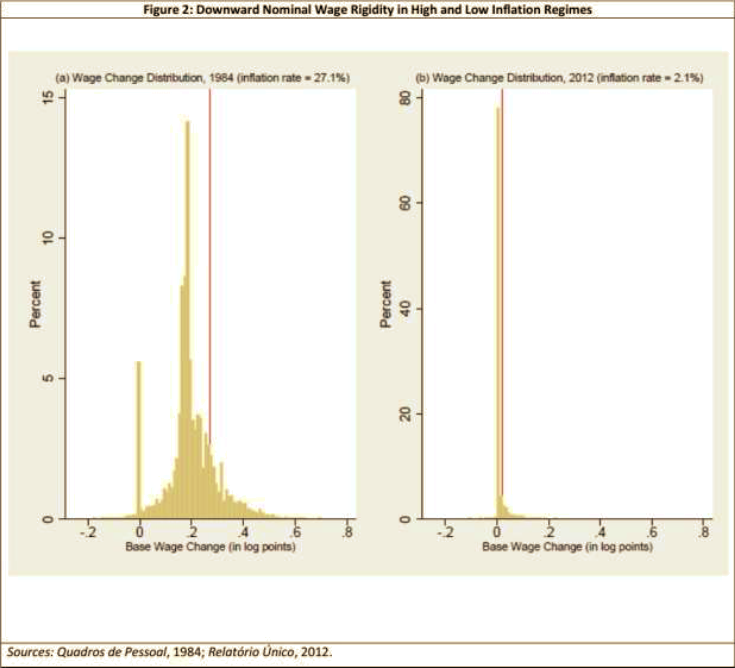
The point here is that while recessions might sometimes simply be the unavoidable result of external factors, the unemployment resulting from recessions is typically caused by our own psychological needs: requiring the numbers in our paychecks to never go down.

The Solution

Once we recognize this fact, the solution to cyclical unemployment is straightforward: more inflation. The issue is that inflation imposes its own costs on society Welfare cost of inflation . Inflation., so we want to find the minimum amount of inflation to keep cyclical unemployment at zero.

Let $w$ be the average wage and let $L$ be the amount of labor done. It follows that total compensation is given by $w \cdot L$.

Now consider total compensation for labor before and after a shock: $w_1 \cdot L_1$ vs $w_2 \cdot L_2$. I want to make two assumptions now:

1. Wages are "sticky" in that they won't go down in the short-run.
2. Employers will never pay their current employees more when they can hire new workers for cheaper.

Now, for the policy I will prove will end cyclical unemployment: The Federal Reserve print enough money so that total labor compensation never goes down. That is, they promise to make it so $w_1 \cdot L_1 \leq w_2 \cdot L_2$.

We know this is within the Fed's power since they can, at worse, increase the money supply by 100x to make wages go up nearly 100-fold.

So, the shock hits and there are two scenarios:

• The Fed just barely does its job and $w_1 \cdot L_1 = w_2 \cdot L_2$. In this case, the only way to get unemployment is if $w_1 \lt w_2$. That is: employers are deliberately raising worker's salaries despite others willing to do the work for the current wage. This contradicts assumption 2.
• The Fed goes beyond the call of duty and $w_1 \cdot L_1 \lt w_2 \cdot L_2$. In this scenario, either (a) unemployment actually goes even lower than pre-shock or (b) wages go up. But, like with the earlier scenario, if wages are going up, we can't have cyclical unemployment.

Finally, note what happens when the Fed fails to perform it's duty. The math becomes $w_1 \cdot L_1 \gt w_2 \cdot L_2$. Since nominal wages can't fall, this causes $L$ to decrease.

This means printing enough money to keep total labor compensation constant is the minimum amount of inflation that can achieve 0 cyclical unemployment.

Some people might find this a little too simple, but what I like most about this justification is that it doesn't rely on any complicated mathematical models or complex, implausible assumptions. No partial derivatives or matrices - just middle school algebra; no hard efficiency requirements or complex economic relationships: just basic employer self-interest.

Caveats

There are a number of caveats with this analysis.

One issue is demographic: if the labor force increases by X% and total compensation remains constant, this implies (assuming sticky wages) an increase in the unemployment rate of 1 percentage point.

This problem is pretty easy to account for: the Fed just has to guarantee total compensation grows at least as fast as the working-age population.

A harder issue is that if total labor compensation stays the same overall, it might be the case that wages are going up in Sector A, but unemployment is surging in Sector B.

This is a challenging problem for which I have no real solution. It's impossible, just looking at the data, to tell whether layoffs in a sector represent an increase in cyclical unemployment, structural unemployment, or just a temporary shift of workers from one sector to another.

That being said,

• It's not obvious to me whether this would be a issue in managing short-term economic fluctuations
• Sumner typically advocates adding ~3 percentage points of wiggle room to the annual growth total, which should smooth over this issue.
• Even if it were, the policy of "never let total compensation fall" would still be a good red-flag: if total compensation ever falls, we *know* the Federal reserve isn't being sufficiently expansionary.

Inadequacy

This all sounds good, and I continue to believe the weak claim that unemployment will go up if total compensation decreases. However, I no long believe the strong claim: that as long as total compensation increases faster than the working-age population, everything will be fine.

What changed my mind? Two things. The first is that total compensation almost never decreases:

If this red flag only triggers once during the deepest recession in the last three generations, it's probably not very useful in real life.

At this point, you might just say that we might be able to make a bit weaker claim: "as long as labor income doesn't fall more than X%, we won't get unemployment." Unfortunately, this is also wrong. During the mid 1970s, we had a recession while inflation was around 10%, which almost certainly means labor income was growing more than 2% per quarter the entire recession.

My opinion echos others that this is because many workers demanded automatic inflation-adjustments to their wages, especially when inflation is high.

This suggests that it's not just sufficiently high (nominal) labor income growth that's important, but also sufficiently stable growth, a point Scott Sumner makes but I hadn't appreciated until now.